Outreach:
In this page you can find links to online talks, and courses as well as some general press articles.
Online Conferences
2022 - Instituto de Estudios Avanzados. Soluciones débiles en Magnetohidrodinámica
Weak Solutions to Ideal Magnetohidrodynamic equations. I will explain how to use the theory of convex integration to Project solutions to the Faraday System to solutions to the full MHD system. As a byproduct we show the existence of critical solutions preserving.
And dissipating magnetic helicity. The second one uses an anisotropic version of staircase laminates.
Homogenization and Inverse Problems
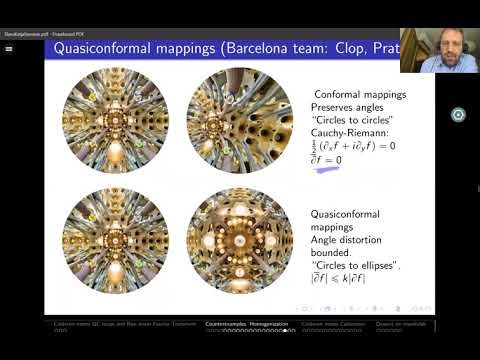
I will review some conditional stability results and recovery algorithms in various inverse problems with irregular coeficients. The arguments use the quasiconformal mappings and a connection with the nonelliptic time dependent Schrödinger equation. Then I will discuss in detail how G-convergence can be utilized to create instabilities in inverse problems. The latter is a work with Y. Kurylev and A. Ruiz. If time allows, I will sketch part of a program with Guijarro, Kurylev and Ruiz to homogenize elliptic equation in paralellizable manifolds.
2021 - International Zoom Inverse Problem
This talk is devoted to the work of Slava Kurylev, our colleague and friend who left us a few years ago. The talk starts with my first work wih Slava on the relation between G-convergence and Dirichlet to Neuman maps and finishing describing our long term project on describing how to obtain explicit formulas in Manifolds.
Convocatoria Divulgativa UAM
Interview by the Foundation of the Universidad Autonoma de Madrid.
Mixing Solutions after Smoothness and Raeleigh-Taylor breakdown. Edimburgh 2021
It is shown that the celebrated solutions to the Muskat Problem by Castro-Cordoba-Gacedo-Fefferman in which the interfase evolves from an analytic graph to an in irregular turned interfase can be extended by convex integration.
PhD course at the Hausdorff Institute in Bonn
This course is an introduction to convex integration. After explaining the basic notions, the core of the course is explaining the impact of this technique in modellling instabilities in fluid mechanis. The model is the Muskat Problem but the strategy is very flexible. In order to constrac macroscopical solutions describing a continuous evolution one has to solve a non linear and non local equation. Th analyisis of such equation is based in adapting the philosophy of microlocal analysis to investigate boundary value problems. The basics of this theory are also discussed.
Artículos de Prensa
- El País - Se explica como la técnica de integración convexa se puede usar para modelar situaciones inestables en mecánica de fluidos.
- El País - Se explican las ecuaciones de la magneto hidrodinámica y su relación con la conjetura de Taylor y las auroras boreales.
- La Vanguardia - Se explica los objetivos del proyecto quamap.
- El confidencial - Se explica la importancia de los proyectos ERC.