In the following examples, c is the number of tones of gray allowed in
dithering.
First of all, let us see the need of dithering. Consider for instance
the following Matlab/Octave program that draws a spherical gradient and
quantize it:
|
% GRADIENT
N = 400;
[X,Y]=meshgrid( linspace(-1,1.01,N), linspace(-1,1.01,N) );
Z = 0.99*sqrt( max(1-(X.^2+Y.^2),0) );
I = mat2gray(Z);
figure(1)
imshow(I)
imwrite(I, 'gradient.png')
|
% QUANTIZATION
c = 14
figure(2)
A = floor( c*Z );
I = mat2gray(A);
imshow(I)
imwrite(I, 'output.png')
|
The gradient is:
and the corresponding quantized images for c=6 and c=14 gray tones are
quite deffective:
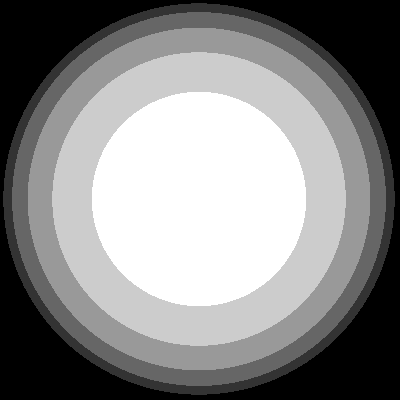 |
 |
c = 6
|
c = 14
|
The most pedestrian way of performing dithering is to apply a random
noise before quantization. These are the results with uniformly
distributed noise at each pixel:
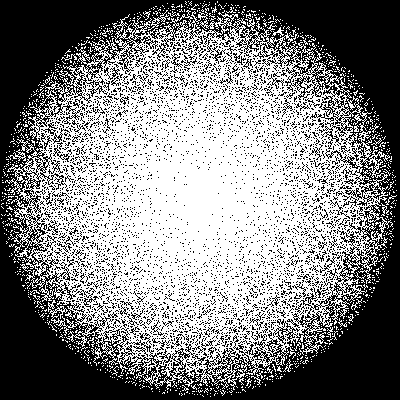 |
 |
c = 2
|
c = 6
|
 |
 |
c = 10
|
c = 14
|
Now we consider the following photo (500x500) as input to appreciate
finer details:
We work with the B/W version.
In ordered dithering, Bayer matrices are employed instead of random
noise to assure the differences of near pixels in the middle tones. The
Bayer matrices for dimension 2k, say Mk, follow a
simple recurrence. Starting by M0=(0), the first block 2k-1x2k-1
is Mk-1, the second (to the right) is Mk-1 + 2·2-2k,
the third is Mk-1 + 3·2-2k and the fourth Mk-1
+ 2-2k.
These are examples with c=4 taking M2 and M4. The
last one is very common in practice.

|
 |
dim = 4, c =
4
|
dim = 16, c
= 4 |
Finally, these are some examples with diffusion dithering, namely with
Floyd-Steinberg algorithm. It distributes the quantization error among
the neighboring pixels with a simple formula. The results are
nevertheless impressive, especially taking into account how easy is to
code it.

|
 |
c = 2
|
c
= 4 |
 |
 |
c = 6
|
c
= 8 |
For more examples and theory on dithering see http://caca.zoy.org/study/index.html.
The code
This is the matlab/octave code employed to generate the images:
% note: octave: pkg load image
% oct = 1 -> octave (matlab otherwise)
oct = 1;
if oct == 1
pkg load image
end
%----------------------------------------------------
% GRADIENT
%----------------------------------------------------
disp(' ')
disp('============================================================')
N = 400;
[X,Y]=meshgrid( linspace(-1,1.01,N), linspace(-1,1.01,N) );
Z = sqrt( max(1-(X.^2+Y.^2),0) );
% Z = matrix with the gradient
I = mat2gray(Z);
imshow(I)
imwrite(I,'../images/ogdith.png');
disp('Gradient saved as "ogdith.png" (400x400)')
disp('============================================================')
%----------------------------------------------------
% TRIVIAL QUANTIZATION
%----------------------------------------------------
for c = 2:4:14
figure(1)
A = floor( (c-1)*Z+0.5 )/(c-1);
I = mat2gray(A);
imshow(I)
namefig = '../images/gndith%d.png';
namefig = sprintf(namefig,c);
imwrite(I,namefig);
end
disp('Fig 1. Trivial quantization saved as "gndith*.png"')
disp('============================================================')
%----------------------------------------------------
% RANDOM NOISE
%----------------------------------------------------
for c = 2:4:14
figure(2)
nois = rand(N)-0.5;
A = floor( (c-1)*Z+nois +0.5)/(c-1);
I = mat2gray(A);
imshow(I)
namefig = '../images/gdith%d.png';
namefig = sprintf(namefig,c);
imwrite(I,namefig);
end
disp('Fig 2. Random noise saved as "gdith*.png"')
disp('============================================================')
%----------------------------------------------------
% PHOTO
%----------------------------------------------------
disp(' ')
disp('============================================================')
imag = imread('../images/zorig_tcol500.jpg');
imag = rgb2gray(imag);
Z = im2double(imag)-0.00000001;
N = size(Z,1);
% Z = matrix with photo
disp('Read photo "zorig_tcol500.jpg" (500x500)')
disp('============================================================')
%----------------------------------------------------
% TRIVIAL QUANTIZATION (P)
%----------------------------------------------------
for c = 2:4:6
figure(3)
A = floor( (c-1)*Z+0.5 )/(c-1);
I = mat2gray(A);
imshow(I)
namefig = '../images/tndith%d.png';
namefig = sprintf(namefig,c);
imwrite(I,namefig);
end
disp('Fig 3. Trivial quantization (P) saved as "tndith*.png"')
disp('============================================================')
%----------------------------------------------------
% RANDOM NOISE (P)
%----------------------------------------------------
for c = 2:4:6
figure(4)
nois = rand(N)-0.5;
A = floor( (c-1)*Z+nois+0.5)/(c-1);
I = mat2gray(A);
imshow(I)
namefig = '../images/tdith%d.png';
namefig = sprintf(namefig,c);
imwrite(I,namefig);
end
disp('Fig 4. Random noise (P) saved as "tdith*.png"')
disp('============================================================')
%----------------------------------------------------
% ORDERED DITHERING
%----------------------------------------------------
M1 = [0,2;3,1]/4;
M2 = [M1,M1+2/16;M1+3/16,M1+1/16];
M3 = [M2,M2+2/64;M2+3/64,M2+1/64];
M4 = [M3,M3+2/256;M3+3/256,M3+1/256];
M5 = [M4,M4+2/1024;M4+3/1024,M4+1/1024];
% only using M = M2 (4x4) and M = M4 (16x16)
for mat = 4:12:16
if mat==4
M = M2;
elseif mat==16
M = M4;
end
Nsub = floor(N/size(M,1)) +1;
DT = repmat(M, 1, Nsub);
DT = repmat(DT, Nsub, 1);
DT = DT(1:N,1:N);
% DT = full size dithering matrix
for c = 2:2:8
figure(5)
A = floor( (c-1)*Z+DT)/(c-1);
I = mat2gray(A);
imshow(I)
namefig = '../images/ord_dith%d_%d.png';
namefig = sprintf(namefig,size(M,1),c);
imwrite(I,namefig)
end
end
disp('Fig 5. Ordered dithering saved as "ord_dith*_*.png"')
disp('============================================================')
%----------------------------------------------------
% FLOYD-STEINBERG DITHERING
%----------------------------------------------------
for c = 2:2:8
figure(6)
A = (c-1)*Z;
for y = 1:N
for x = 1:N
oldpixel = A(x,y);
newpixel = round(oldpixel);
A(x,y) = newpixel;
quant_error = oldpixel - newpixel;
if x<N
A(x + 1,y)= A(x + 1,y) + quant_error * 7 / 16;
end
if ( x>1 ) && ( y<N )
A(x - 1,y + 1) = A(x - 1,y + 1) + quant_error * 3 / 16;
end
if y<N
A(x ,y + 1) = A(x ,y + 1) + quant_error * 5 / 16;
end
if ( x<N ) && ( y<N )
A(x + 1,y + 1) = A(x + 1,y + 1) + quant_error * 1 / 16;
end
end
end
I = mat2gray(A);
imshow(I)
namefig = '../images/dif_dith%d.png';
namefig = sprintf(namefig,c);
imwrite(I,namefig)
end
disp('Fig 6. Floyd-Steinberg dithering saved as "dif_dith*.png"')
disp('============================================================')














